Temperature-to-period circuit provides linearization of thermistor response

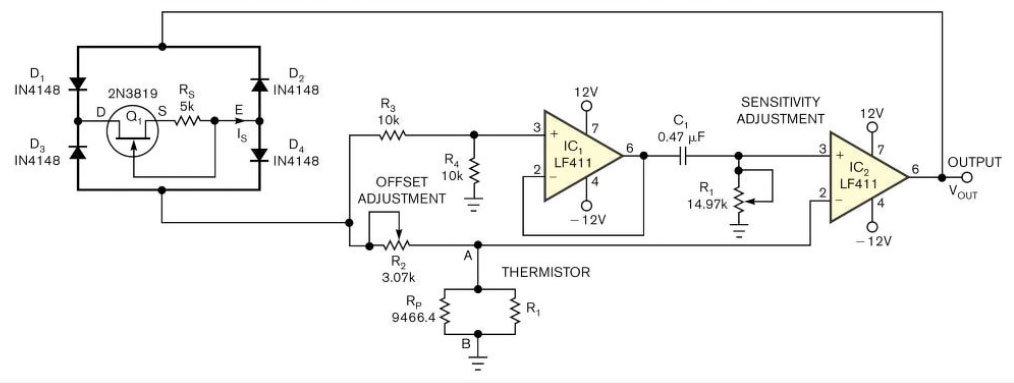
Designers often use thermistors rather than other temperature sensors because thermistors offer high sensitivity, compactness, low cost, and small time constants. But most thermistors’ resistance-versus-temperature characteristics are highly nonlinear and need correction for applications that require a linear response. Using a thermistor as a sensor, the simple circuit in Figure 1 provides a time period varying linearly with temperature with a nonlinearity error of less than 0.1K over a range as high as 30K. You can use a frequency counter to convert the period into a digital output. An approximation derived from Bosson’s Law for thermistor resistance, R_T, as a function of temperature, θ, comprises R_T=AB^–θ (see sidebar “Exploring Bosson’s Law and its equation”). This relationship closely represents an actual thermistor’s behavior over a narrow temperature range.

Figure 1 This simple circuit linearizes a thermistor’s response and produces an output period that’s proportional to temperature.

You can connect a parallel resistance, R_P, of appropriate value across the thermistor and obtain an effective resistance that tracks fairly close to AB^–θ 30K. In Figure 1, the network connected between terminals A and B provides an effective resistance of R_AB AB^–θ. JFET Q₁ and resistance R_S form a current regulator that supplies a constant current sink, I_S, between terminals D and E.

Through buffer-amplifier IC₁, the voltage across R₄ excites the RC circuit comprising R₁ and C₁ in series, producing an exponentially decaying voltage across R₁ when R₂ is greater than R_AB. At the instant when the decaying voltage across R₁ falls below the voltage across thermistor R_T, the output of comparator IC₂ changes its state. The circuit oscillates, producing the voltage waveforms in Figure 2 at IC₂‘s output. The period of oscillation, T, is T=2R₁C₁ln(R₂/R_AB)2R₁C₁[ln(R₂/A)+θlnB]. This equation indicates that T varies linearly with thermistor temperature θ.

Read more: Temperature-to-period circuit provides linearization of thermistor response